• University of Waterloo

• MTE MISC

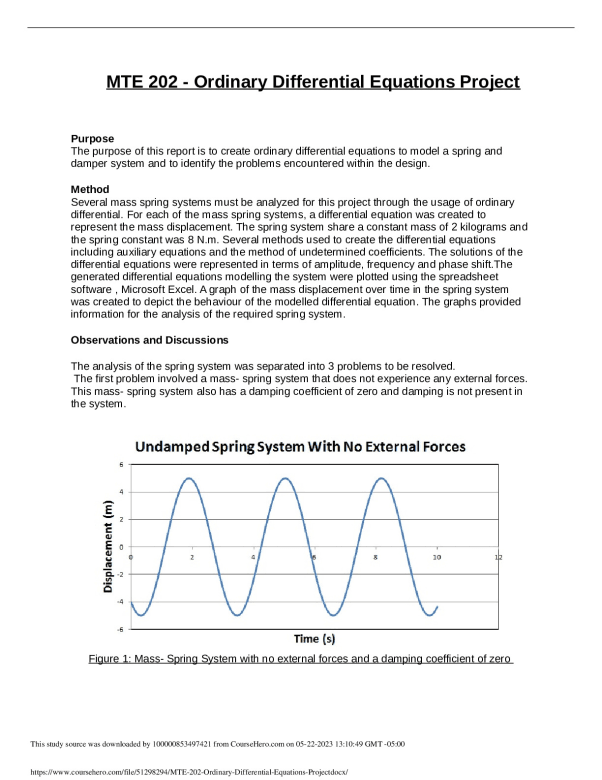
 MTE 202 - Ordinary Differential Equations Project Purpose The purpose of this report is to create ordinary differential equations to model a spring and damper system and to identify the problems encountered within the design. Method Several mass spring systems must be analyzed for this project through the usage of ordinary differential. For each of the mass spring systems, a differential equation was created to represent the mass displacement. The spring system share a constant mass of 2 kilograms and the spring constant was 8 N.m. Several methods used to create the differential equations including auxiliary equations and the method of undetermined coefficients. The solutions of the differential equations were represented in terms of amplitude, frequency and phase shift.The generated differential equations modelling the system were plotted using the spreadsheet software , Microsoft Excel. A graph of the mass displacement over time in the spring system was created to depict the behaviour of the modelled differential equation. The graphs provided information for the analysis of the required spring system. Observations and Discussions The analysis of the spring system was separated into 3 problems to be resolved. The first problem involved a mass- spring system that does not experience any external forces. This mass- spring system also has a damping coefficient of zero and damping is not present in the system. Figure 1: Mass- Spring System with no external forces and a damping coefficient of zero This study source was downloaded by 100000853497421 from CourseHero.com on 05-22-2023 13:10:49 GMT -05:00 https://www.coursehero.com/file/51298294/MTE-202-Ordinary-Differential-Equations-Projectdocx/ The graph shows the displacement time curve, for the general equation derived from the differential equation: The only two forces acting in the first case are” ma” and the spring force. The damping coefficient for the first equation is zero, hence a graph with a constant amplitude was expected, since there was no damping involved. A sinusoidal wave is obtained with an amplitude of 5 and a phase shift angle of 0.93 radians. The graph satisfies the initial condition, showing that the total displacement at time ‘0’ is -4. There was no external force applied on the system in the first case, hence the graph shows a constant amplitude and frequency. The general equation obtained in terms of amplitude and phase shift is y = 5 Sin(2t+0.923). Hence the curve has an amplitude of 5 and a phase shift angle of 0.923 radians. If a stiffer spring is in the mass- spring system, the expected result would have a smaller displacement in the mass. The frequency of the vibrations is expected to increase with a stiffer spring due to the lower amplitude. The phase shift of the system is expecte